In his celebrated book Personal Knowledge, the renowned physical chemist and philosopher Michael Polanyi made the case for the scientist’s personal participation in knowledge-discovery and knowledge-validation. Whilst our culture commonly prizes scientific objectivity and detachment, for Polanyi, ‘knowing’ is a deeply personal act (and art), melding skill, commitment, and passionate engagement with the chosen domain.
In making his case, Polanyi takes a deep delve into the nature of skills, and begins with the observation (which he terms ‘a fact’) that “… a skilful performance is achieved by the observance of a set of rules which are not known as such to the person following them”. By way of example, from his interrogations of physicists, engineers and bicycle manufacturers, he came to the conclusion that the principle by which cyclists keep their balance “is not generally known”. For what it’s worth, here is the rule observed by cyclists:
When falling to the right they turn the handlebars to the right, so that the course of the bike is deflected along a curve towards the right. This results in a centrifugal force pushing the cyclist to the left and offsets the gravitational force dragging them down to the right. This manoeuvre throws the cyclist out of balance to the left, which they counteract by turning the handlebars to the left, and so they keep in balance by winding along a series of appropriate curvatures. For a given angle of imbalance the curvature of each winding is inversely proportional to the square of the speed at which the cyclist is proceeding.
Of course no-one learns to ride a bike by first learning this rule. And if they did, they’d fail, as a number of other factors – crucial in practice – are omitted in its formulation. Beyond the barest of instructions, we learn to ride a bike by riding a bike. And we learn to play chess by playing chess – and that’s as true for AlphaZero as it is for us. For Polanyi, “… rules of art can be useful, but they do not determine the practice of an art; they are maxims, which can serve as a guide to an art only if they can be integrated into the practical knowledge of the art. They cannot replace this knowledge.”
Implicit learning
It is this integration of the rule and the practical knowledge that comprises what we commonly refer to as ‘tacit knowledge’ and ‘implicit learning’. By its very definition, implicit learning is the learning of complex information in an incidental manner, without awareness of what has been learned (Sun, R., 2008; The Cambridge Handbook of Computational Psychology). Implicit learning happens, therefore, when we’re not consciously aware of it happening. Though learning can be accelerated and deepened and made explicit by ramping up our metacognitive toolset (to get us thinking about our thinking), it’s going to happen at a certain level anyway, simply through experience – watching, listening, imitating, and experimenting – just as we all learned once to speak and to walk. And, as adults, just as we accommodate to the customs, expectations, and practices of a new work or office culture even in the absence of a formal set of guidelines.
A frequent query from Chessable users relates to their being asked to repeat a move straight after they’ve seen it for the first time. What’s the challenge? Where’s the learning? What’s the point of this apparently inane, mindless act? The answer to these legitimate questions lies in the notion of implicit learning: we watch a move being played, we imitate it (immediately) and practise it (in review mode), and we experiment with it in our own games, online or over the board. Move Trainer plays the coach-role that our parents played intuitively when we were infants. It encourages us, supports us and holds us accountable to do better when we fail. And in the process, we learn.
Just do it!
There is a closely related question that can also be asked: why am I sometimes asked to make a move in the absence of an explicit explanation? Assuming no inadvertent omission on the part of the course creator, the answer again lies in the area of implicit learning. I like Polanyi’s use of the word ineffable to describe those moments when explicit articulation would be impossible: “The area where the tacit predominates to the extent that articulation is virtually impossible; we may call this the ineffable domain”. In a conversation with the filmmaker (and chessplayer) Alan Byron, Magnus Carlsen claimed that he couldn’t explain how or why he played chess so well – “I just do”. Not overly helpful pedagogically, perhaps, but an honest expression of the ineffable.
Because this domain includes all those things that can’t easily be expressed or codified or articulated or transferred to others, we often take the easy way out and dismiss things simply as lumpen intuition. But that which we term intuition, and to which we often attribute a slightly mystical provenance (like ‘natural talent’), can be traced to the learnings accruing from the steady accretion of practical experience: Keith Arkell’s expertise in rook endings, for instance, or Mickey ‘The Spider’ Adam’s ‘sixth sense’ for where pieces belong on a board (many moves before they’re actually needed there), or Vladimir Kramnik’s gift for knowing what to do when he doesn’t know what to do. It’s sometimes possible to get close to articulating the processes by which this knowledge is gained and exercised – and both Arkell in his recent book (Arkell’s Endings) and Kramnik in his first Chessable course set out explicitly to do this – but as Polanyi reminds us, these resources cannot substitute for their conversion from maxim to action: we must also strive to enact this knowledge for ourselves.
Intuition in chess praxis
I’ve been reminded personally of the source and nature of implicit learning, personal knowledge and intuition in a recent exchange with GM Peter Wells. Here’s the context: my joyous return to over the board play took place in early July, whilst en vacance in southwest France. In round 4 of the Hourtin Wine Open I had the chance to unleash a beautiful combination against an opponent rated exactly 350 points higher than me:
B. Hymer – T. Jahn, Hourtin 2021
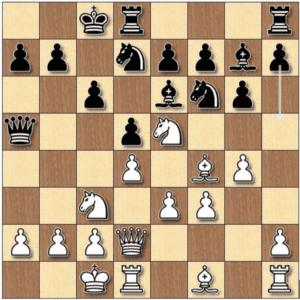
In this position, with a raking dark-squared bishop on f4 and with the benefit of having Crafty Raf’s Mating Patterns course under my belt, I spotted the possibility of opening the a6-c8 diagonal to deadly effect by Nxc6, possibly in combination with an embarrassment of the loose black queen on a5 by Nxd5. It doesn’t work immediately of course, so I considered the preparatory 11 Qd3, but this blatant signalling of my intentions would surely have resulted in a simple exchange of knights on e5, and a sheepishly placed queen of my own. Instead I played the sly 11 Kb1!?, which prophylaxis gives fewer clues as to my aggressive intentions. My opponent missed the threat completely, failed to exchange knights on e5, and instead played the natural-looking 11 … h5(?).
It’s on! With my racing heartbeat audible to everyone in the playing hall, I checked my lines: 12 Nxc6! bxc6 13 Nxd5! Qxd2 14 Ba6#, and gold coins will rain down on the board. But what’s this? What if instead of 13 … Qxd2 Black just plays his queen to a4, to keep an eye on the a6 square? 14 b3 Qa3 achieves little, and I don’t have enough for my piece. Nothing else presented itself to me, so instead I launched Plan B – the immediate 12 Nxd5. The queen can’t capture the knight without being ensnared, but amazingly after 12 … Qxd2 13 Nxe7+ Kb8 14 Nxd7+ Ka8 my knights aren’t long for this world and the big chance had gone. In desperation and confusion I chased down an illusory mate on the a-file by saccing a knight on b6 and then capturing the queen on d2 en route to a3, but of course found that square denied to me by … Bf8. Sickened by the sense that I must have missed a glorious win somewhere, I crumbled in short order.
Only after the game did I spot the beautiful and winning line, 12 Nxc6! bxc6 13 Nxd5! Qa4 14 Qb4! (the right deflection) … Qxb4 15 Ba6+ Qb7 16 Nxe7#.
Reflecting on this missed opportunity, I was struck by Peter’s comment:
“I increasingly find myself looking at psychological explanations for such things these days (since there is no doubt that 14 Qb4 should be and is quite within your capabilities). I wonder whether there is something which causes us to be sceptical of such tactics working precisely because they are so beautiful? To me 13…Qa4 feels like it should be teetering on the brink (as it is), but I think it is all too easy to dismiss something like 12 Nxc6 as ‘fantasy’ and therefore to be unduly generous to ‘resources’ such as 13…Qa4 with a narrative which almost nags us with the thought that ‘such beautiful possibilities don’t happen to us in real life’. Does that ring true at all?”
It does, but on the theme of implicit learning I am drawn especially to the line, “13…Qa4 feels like it should be teetering on the brink (as it is)”: an experienced GM, with a lifetime’s immersion in the game, is paying homage to the ineffable – a pre-concrete feeling that something shouldn’t work; a hunch; an intuition, if you will. This despite being known for many precisely-calculated and aesthetically gorgeous combinations in his time. It is this feeling that would have alerted Peter to the need to invest energy in finding the concrete justification, the move that causes the teetering black position finally to topple.
Trusting the process
Time and time again the science of learning reminds us to treat the products of learning not as the primary object of our desires, but as the secondary gifts of an intense fixation on the processes. Not everything that is implicitly true can be explicitly articulated or verified. There is a place for objective rationality for sure, but sometimes there is a space for gut feel. Not every move can be played with full understanding of the consequences – there is a space for ‘reasonable doubt’. And sometimes, in pursuit of chess improvement, we may not ‘understand’ why we’re being asked to play a move at all. But when we follow wise processes of learning, in the long run the products reveal themselves too. And what is more, these processes can and should be the source of the greatest pleasure. As the Swiss mathematician Leonhard Euler wrote in 1808, “It is not knowledge, but the act of learning, not possession but the act of getting there, which grants the greatest enjoyment”. Should I seek to put this into practice myself? That’s implicit!